SUVAT equations form the foundation of kinematics in physics. These equations describe motion under constant acceleration and are essential for solving problems related to velocity, displacement, and time. Understanding how to apply SUVAT equations correctly is crucial for students, engineers, and scientists working in fields such as mechanics, aerospace, and automotive engineering.
This guide provides a complete breakdown of the five SUVAT equations, their derivations, real-world applications, common problem-solving techniques, interactive learning strategies, and frequent mistakes students make.
Understanding SUVAT Equations
What is SUVAT?
SUVAT equations describe motion when acceleration is uniform. The name SUVAT is derived from five key motion variables:
- S = Displacement (m)
- U = Initial velocity (m/s)
- V = Final velocity (m/s)
- A = Acceleration (m/s²)
- T = Time (s)
These equations are applicable only when the acceleration remains constant throughout the motion.
List of SUVAT Equations and Their Applications
| Equation | Formula | Use Case |
|---|---|---|
| First | v=u+atv = u + atv=u+at | Finding final velocity when time and acceleration are known |
| Second | s=ut+12at2s = ut + \frac{1}{2}at^2s=ut+21at2 | Finding displacement when time is given |
| Third | v2=u2+2asv^2 = u^2 + 2asv2=u2+2as | Finding velocity without time |
| Fourth | s=vt−12at2s = vt – \frac{1}{2}at^2s=vt−21at2 | Finding displacement without initial velocity |
| Fifth | s=(u+v)2×ts = \frac{(u+v)}{2} \times ts=2(u+v)×t | Finding displacement with average velocity |
Each equation is useful depending on the given data and what is required to be calculated.
Step-by-Step Problem-Solving Approach
Step 1: Identify the Given Values
Before choosing an equation, extract the known variables from the problem statement and determine which variable needs to be found.
Step 2: Select the Appropriate Equation
Use the table above to match the equation with the given variables and the unknown value.
Step 3: Solve the Equation
- Substitute the known values into the selected equation.
- Ensure unit conversions are correct (e.g., converting km/h to m/s).
- Calculate the result step by step.
Step 4: Verify the Answer
- Cross-check calculations for errors.
- Consider whether the answer is realistic based on the problem context.
Example Problems with Step-by-Step Solutions
Example 1: Finding Final Velocity
Problem: A car accelerates from rest at 333 m/s² for 555 seconds. What is its final velocity?
Solution:
- Given: u=0u = 0u=0, a=3a = 3a=3, t=5t = 5t=5
- Using v=u+atv = u + atv=u+at:v=0+(3×5)v = 0 + (3 \times 5)v=0+(3×5)
v=15 m/sv = 15 \text{ m/s}v=15 m/s
Example 2: Finding Displacement
Problem: A ball is thrown with an initial velocity of 101010 m/s and an acceleration of 222 m/s² for 444 seconds. What is the displacement?
Solution:
- Given: u=10u = 10u=10, a=2a = 2a=2, t=4t = 4t=4
- Using s=ut+12at2s = ut + \frac{1}{2}at^2s=ut+21at2:s=(10×4)+12(2×42)s = (10 \times 4) + \frac{1}{2} (2 \times 4^2)s=(10×4)+21(2×42)
s=40+16=56 ms = 40 + 16 = 56 \text{ m}s=40+16=56 m
Common Mistakes and How to Avoid Them
Mistakes to Avoid
- Selecting the wrong equation for the given data
- Failing to convert units correctly (e.g., km/h to m/s)
- Not considering negative acceleration (deceleration)
- Rounding off calculations too early, leading to incorrect final results
Best Practices for Problem-Solving
- Always list out the known values clearly before selecting an equation.
- Double-check the final units of measurement.
- Use estimation to check whether the answer is reasonable.
- Practice with different types of problems to improve accuracy and speed.
Real-World Applications of SUVAT Equations
Sports Science
SUVAT equations are used to analyze sprint acceleration, track cycling performance, and determine the motion of balls in sports like football and cricket.
Automotive Engineering
Engineers use SUVAT to calculate stopping distances, acceleration rates of vehicles, and braking forces required for safety measures.
Space Science
SUVAT equations help determine the velocity changes required for spacecraft maneuvers and calculate free-fall acceleration on different planets.
Projectile Motion
SUVAT is essential in calculating trajectories for projectiles in military defense, video game physics, and scientific experiments involving parabolic motion.
Interactive Learning Features for SUVAT
Decision-Making Guide for Choosing the Right Equation
Many students struggle to determine which SUVAT equation to use. A structured decision-making flowchart can help:
- Identify the given variables.
- Determine which variable is missing.
- Use a flowchart to decide which equation best fits the problem.
Online SUVAT Calculator for Quick Solutions
Manually solving equations can be time-consuming. Using an SUVAT Calculator simplifies the process:
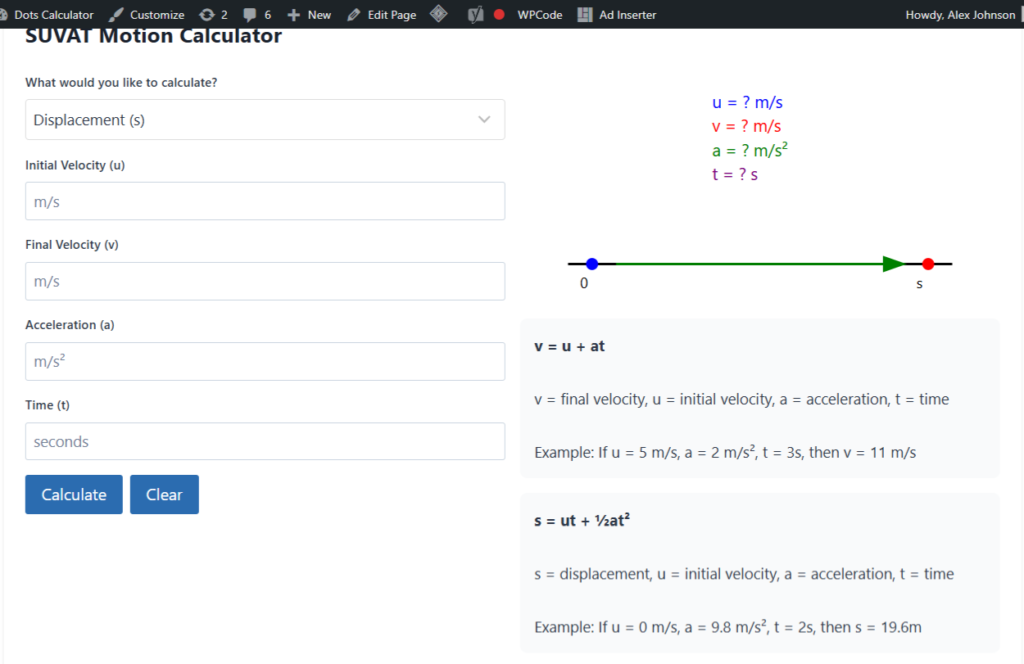
- Input known values
- Automatically determines the correct equation
- Provides step-by-step breakdown of the solution
Access the SUVAT Calculator to instantly solve problems.
Advanced Learning Resources and Study Materials
Interactive Quizzes and Practice Problems
- Multiple-choice questions covering different difficulty levels
- Step-by-step solutions for each practice problem
- Timed quizzes for exam preparation
SUVAT Equations Quiz
Study Guides and External Resources
- Downloadable PDF guides with summarized SUVAT concepts
- Links to video explanations for deeper understanding
- References to research papers and physics forums
Conclusion
Mastering SUVAT equations is essential for solving motion-related physics problems efficiently. Understanding how to apply these equations correctly will enhance problem-solving skills in fields like mechanics, automotive engineering, space science, and sports analysis.
To further solidify your understanding, use interactive tools like the SUVAT calculator, attempt practice quizzes, and review real-world case studies. Consistent practice and familiarity with different problem types will lead to mastery of these fundamental physics equations.
FAQs
How do I know which SUVAT equation to use?
- Identify the known values and the unknown variable.
- Choose the equation that includes everything except the missing variable.
How do I determine the correct SUVAT equation to use in an exam question?
In A-Level exams, start by identifying the known variables (e.g., U, V, A, S, T) and the one you need to calculate. For example, if time (T) is not given, use the equation:
V ² = U ²+ 2AS
Practice is essential to quickly match the problem to the correct equation during exams.
Why is the equation V² = U² + 2AS frequently used in A-Level mechanics?
This equation is particularly useful for problems where time is not provided. It allows you to calculate the final speed of an object or the displacement needed to reach a specific velocity. It is derived by combining the definitions of acceleration and displacement, excluding the time variable.
How are SUVAT equations applied in practical A-Level Physics problems?
In A-Level Physics, these equations are widely used in scenarios such as:
- Free-fall motion under gravity.
- Motion on inclined planes.
- Vehicle acceleration and deceleration.
They are applicable for solving both vertical and horizontal motion problems as long as the acceleration is constant.
Can SUVAT equations be used for vertical motion?
Yes, they work for vertical motion, with acceleration due to gravity taken as -9.81 m/s².
Can SUVAT equations be used for non-uniform acceleration?
- No, these equations only apply when acceleration is constant. For variable acceleration, calculus-based methods are required.
How can I improve my problem-solving skills with SUVAT equations?
Practice different types of problems, focus on understanding concepts rather than memorization, and seek guidance from tutors if needed.
What is the best way to practice SUVAT problems?
Use a combination of solved examples, practice quizzes, and real-world applications to develop a strong conceptual understanding. An SUVAT calculator can also be helpful for checking answers.